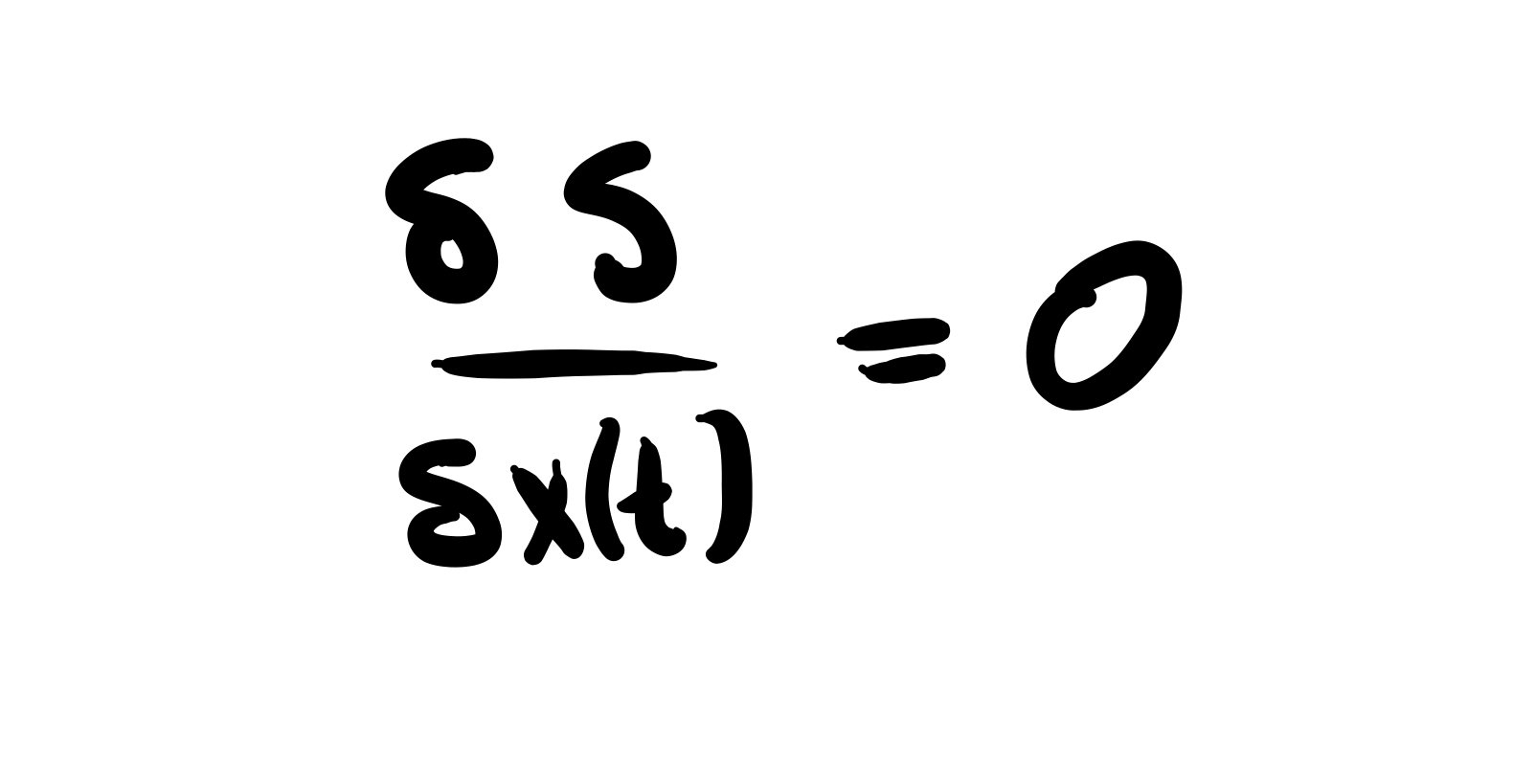Functional Derivative Cheat Sheet
(Update: LaTeX version of the cheat sheet available for download!)
I’ve been rather perplexed at how difficult it is to find good information about functional derivatives on the internet. Many, many textbooks discuss variational calculations––for example, the principle of least action––using what’s called the total variation of a functional:
These are extensively documented (see, e.g., Boas, Mathematical Methods in the Physical Sciences) and so I will not discuss them further here.
However, often in physics one often comes across expressions such as this:
These “functional derivatives” are very similar to the total variation above, but for some reason I have had a much harder time finding good information about them online. When they are discussed, they are often simply treated using the total variation.
However, this is a perfectly well-defined derivative, and it is often quite convenient (and conceptually simpler) to use this form. For this reason, I have thrown together a cheat sheet of functional derivative identities (including the definition, boxed in blue) which are quite useful.
These are all derived carefully here, including many worked examples. But for quick use, I think this will be valuable to people!
Cheers, and happy differentiating!
-Matt